Today, in the latest edition of Math with Margo, converting fractions into decimals is a breeze! (Check out last lesson where we went over a classic algebra practice problem and learned how to solve for x.)
In this simple tutorial, our resident math expert will walk you through an easy-to-follow example!
How to convert fractions into decimals
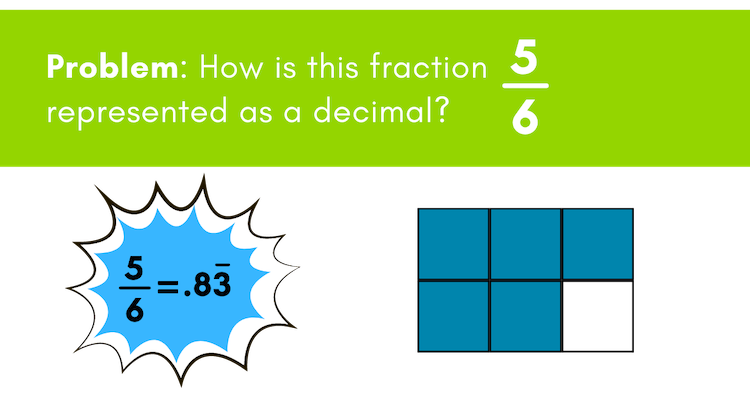
Problem: How is the fraction ⅚ represented as a decimal?

Step One: Write out the problem
The fraction bar is also known as division, so this can be read as 5 divided by 6.

Step Two: Divide the fraction
Since 6 does not go into 5, we must add a decimal and a 0 after 5.
5 and 5.0 are equivalent. Just like saying $5 or $5.00, they are both equal in value. You can add a decimal and as many 0s, and it still won’t change its value.
Now ask yourself, how many times does 6 go into 50? 8 is correct, since 6 times 8 equals 48, which is as close as you can get to 50 without going over.
(Make sure you bring the decimal straight up.)
Learn more: Easy Division Tips & Tricks


Step 3: Address the remainder
Since we have a remainder of 2, we need to add another 0 to the end of 5.0 and bring the 0 down.

Now ask yourself, how many times does 6 go into 20? 3 is correct, since 3 x 8 = 18, which is as close as you can get to 20 without going over.

Step Four: Identify the repeating remainder

You might notice a pattern forming. You are right!
You will continually get a remainder of 2, and instead of writing 3 over and over again, we place a bar over the number(s) that are repeating.

In this case, only the 3 is repeating, so we write our final answer as (above).
Step Five: Write your final answer as a fraction

This means that ⅚ = .83. This makes sense since ⅚ is close to one whole, and . 83 is also close to one whole.
Congratulations! You did it. Wondering how to convert decimals into fractions? We’ve got you covered in this related post.
For more math resources, check out our one on one math tutoring opportunities!
